
For example, it is known that in the trapezoid ABCD, AD∥BC, AB=AD BC, and E is the midpoint of CD. Prove: AE and BE bisect ∠BAD and ∠ABC respectively.
We can start from the known conditions and follow the following steps to draw a figure that meets the meaning of the question on the geometric sketchpad.
Open the geometric sketchpad, use the [Point Tool] to draw two points A and B in the blank space of the sketchpad, select the [Ray Tool] to draw horizontal rays through points A and B respectively, and select points A and B to construct line segments. .
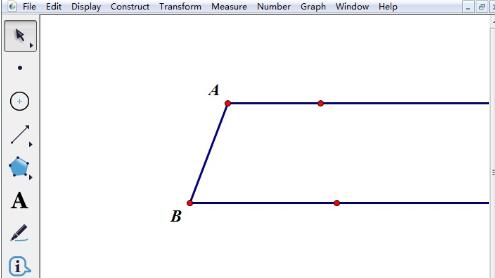
Use the [Point Tool] to pick a point F on the line segment AB, take points A and B as the center points, and use the lengths AF and BF as the radii to draw a circle with the two bases The ray intersects at points D and C. Obviously, AD BC=AB.
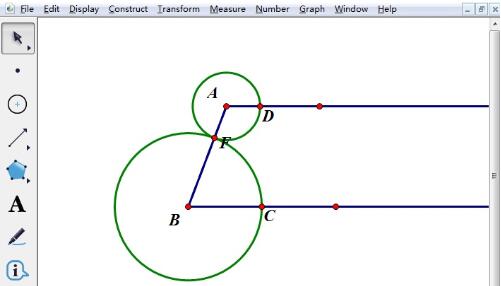
Take the midpoint E of the line segment CD and connect AE and BE. Select points C and D, execute the [Construct]-[Midpoint] command to draw the midpoint E, select points A, E, B, and E in sequence, execute the [Construct]-[Line Segment] command to get the line segments AE, BE.
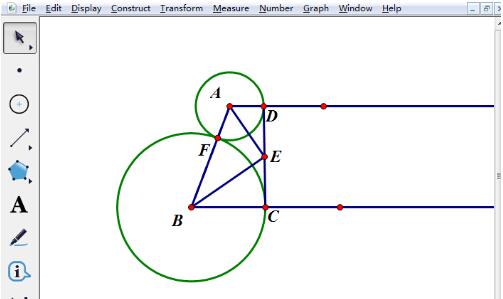
Hide the auxiliary graphics during the drawing process to get graphics that meet the requirements of the question. Select the unnecessary rays and circles and press the shortcut key [Ctrl H] to hide the unnecessary objects and obtain a graphic that combines the meaning of the question as shown in the figure.
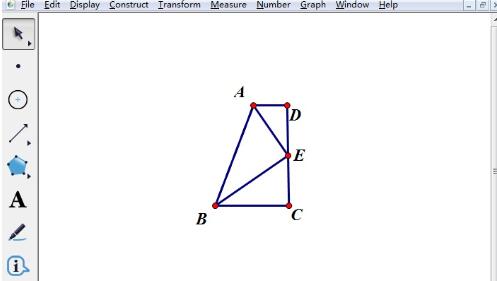
The above is the detailed content of How to draw geometric figures according to known conditions in the geometric sketchpad. For more information, please follow other related articles on the PHP Chinese website!




