Tutoriel détaillé sur le dessin de graphiques tridimensionnels en python

Cet article vous apporte des connaissances pertinentes surPython. Habituellement, nous utilisons Python pour dessiner des diagrammes plans en deux dimensions, mais parfois nous devons également dessiner des diagrammes de scène en trois dimensions. Ce qui suit présente les informations pertinentes sur Python pour dessiner en trois dimensions. diagrammes. J'espère que cela aide tout le monde.

【Recommandation associée :Tutoriel vidéo Python3】

Cet article ne résume que les méthodes de dessin les plus élémentaires.

1. Initialisation

On suppose que le package d'outils matplotlib a été installé.

Utilisez matplotlib.figure.Figure pour créer un cadre :

import matplotlib.pyplot as plt from mpl_toolkits.mplot3d import Axes3D fig = plt.figure() ax = fig.add_subplot(111, projection='3d')

2. Graphiques linéaires

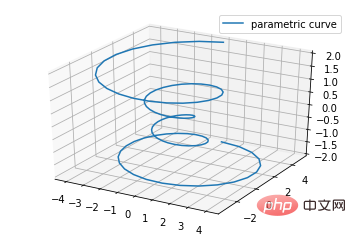
Utilisation de base :

ax.plot(x,y,z,label=' ')

code :

import matplotlib as mpl from mpl_toolkits.mplot3d import Axes3D import numpy as np import matplotlib.pyplot as plt mpl.rcParams['legend.fontsize'] = 10 fig = plt.figure() ax = fig.gca(projection='3d') theta = np.linspace(-4 * np.pi, 4 * np.pi, 100) z = np.linspace(-2, 2, 100) r = z**2 + 1 x = r * np.sin(theta) y = r * np.cos(theta) ax.plot(x, y, z, label='parametric curve') ax.legend() plt.show()

3. Nuages de points (graphiques à nuages de points)

ax.scatter(xs, ys, zs, s=20, c=None, depthshade=True, *args, *kwargs)

xs,ys,zs : données d'entrée ;
s : taille du point de dispersion
c : couleur, telle que c = 'r' est rouge ; Vrai, Faux est opaque
*args, etc. sont des variables étendues, telles que maker = 'o', alors le résultat scatter a la forme de 'o'
• code:

from mpl_toolkits.mplot3d import Axes3D import matplotlib.pyplot as plt import numpy as np def randrange(n, vmin, vmax): ''' Helper function to make an array of random numbers having shape (n, ) with each number distributed Uniform(vmin, vmax). ''' return (vmax - vmin)*np.random.rand(n) + vmin fig = plt.figure() ax = fig.add_subplot(111, projection='3d') n = 100 # For each set of style and range settings, plot n random points in the box # defined by x in [23, 32], y in [0, 100], z in [zlow, zhigh]. for c, m, zlow, zhigh in [('r', 'o', -50, -25), ('b', '^', -30, -5)]: xs = randrange(n, 23, 32) ys = randrange(n, 0, 100) zs = randrange(n, zlow, zhigh) ax.scatter(xs, ys, zs, c=c, marker=m) ax.set_xlabel('X Label') ax.set_ylabel('Y Label') ax.set_zlabel('Z Label') plt.show()

Copier après la connexion

4. Bloc de ligne diagramme (tracés filaires)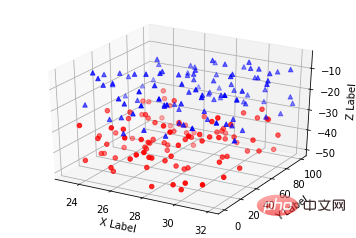

ax.plot_wireframe(X, Y, Z, *args, **kwargs)

Copier après la connexion

X, Y, Z : données d'entrée

rstride : longueur de pas de ligne
cstride : longueur de pas de colonne
rcount : limite supérieure du numéro de ligne
ccount : supérieur limite du numéro de colonne
• code:

from mpl_toolkits.mplot3d import axes3d import matplotlib.pyplot as plt fig = plt.figure() ax = fig.add_subplot(111, projection='3d') # Grab some test data. X, Y, Z = axes3d.get_test_data(0.05) # Plot a basic wireframe. ax.plot_wireframe(X, Y, Z, rstride=10, cstride=10) plt.show()

Copier après la connexion

5. Tracés de surface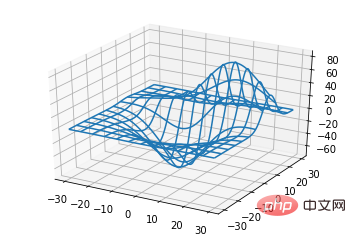

ax.plot_surface(X, Y, Z, *args, **kwargs)

Copier après la connexion

X, Y, Z: données

rstride, cstride, rcount, ccount: Identique à la définition des tracés filaires
couleur : couleur de la surface
cmap : couche
• code :

from mpl_toolkits.mplot3d import Axes3D import matplotlib.pyplot as plt from matplotlib import cm from matplotlib.ticker import LinearLocator, FormatStrFormatter import numpy as np fig = plt.figure() ax = fig.gca(projection='3d') # Make data. X = np.arange(-5, 5, 0.25) Y = np.arange(-5, 5, 0.25) X, Y = np.meshgrid(X, Y) R = np.sqrt(X**2 + Y**2) Z = np.sin(R) # Plot the surface. surf = ax.plot_surface(X, Y, Z, cmap=cm.coolwarm, linewidth=0, antialiased=False) # Customize the z axis. ax.set_zlim(-1.01, 1.01) ax.zaxis.set_major_locator(LinearLocator(10)) ax.zaxis.set_major_formatter(FormatStrFormatter('%.02f')) # Add a color bar which maps values to colors. fig.colorbar(surf, shrink=0.5, aspect=5) plt.show()

Copier après la connexion

6. Tracés tri-surface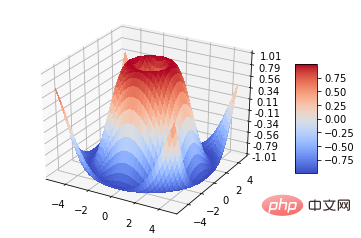

ax.plot_trisurf(*args, **kwargs)

Copier après la connexion

X, Y, Z : données

Les autres paramètres sont similaires au tracé de surface
• code:

from mpl_toolkits.mplot3d import Axes3D import matplotlib.pyplot as plt import numpy as np n_radii = 8 n_angles = 36 # Make radii and angles spaces (radius r=0 omitted to eliminate duplication). radii = np.linspace(0.125, 1.0, n_radii) angles = np.linspace(0, 2*np.pi, n_angles, endpoint=False) # Repeat all angles for each radius. angles = np.repeat(angles[..., np.newaxis], n_radii, axis=1) # Convert polar (radii, angles) coords to cartesian (x, y) coords. # (0, 0) is manually added at this stage, so there will be no duplicate # points in the (x, y) plane. x = np.append(0, (radii*np.cos(angles)).flatten()) y = np.append(0, (radii*np.sin(angles)).flatten()) # Compute z to make the pringle surface. z = np.sin(-x*y) fig = plt.figure() ax = fig.gca(projection='3d') ax.plot_trisurf(x, y, z, linewidth=0.2, antialiased=True) plt.show()

Copier après la connexion

7. Tracés de contour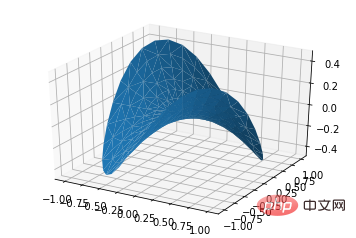

ax.contour(X, Y, Z, *args, **kwargs)

Copier après la connexion

from mpl_toolkits.mplot3d import axes3d import matplotlib.pyplot as plt from matplotlib import cm fig = plt.figure() ax = fig.add_subplot(111, projection='3d') X, Y, Z = axes3d.get_test_data(0.05) cset = ax.contour(X, Y, Z, cmap=cm.coolwarm) ax.clabel(cset, fontsize=9, inline=1) plt.show()

Contours bidimensionnels, il peut également être dessiné avec un dessin tridimensionnel carte de surface :

from mpl_toolkits.mplot3d import axes3d from mpl_toolkits.mplot3d import axes3d import matplotlib.pyplot as plt from matplotlib import cm fig = plt.figure() ax = fig.gca(projection='3d') X, Y, Z = axes3d.get_test_data(0.05) ax.plot_surface(X, Y, Z, rstride=8, cstride=8, alpha=0.3) cset = ax.contour(X, Y, Z, zdir='z', offset=-100, cmap=cm.coolwarm) cset = ax.contour(X, Y, Z, zdir='x', offset=-40, cmap=cm.coolwarm) cset = ax.contour(X, Y, Z, zdir='y', offset=40, cmap=cm.coolwarm) ax.set_xlabel('X') ax.set_xlim(-40, 40) ax.set_ylabel('Y') ax.set_ylim(-40, 40) ax.set_zlabel('Z') ax.set_zlim(-100, 100) plt.show()

Il peut également s'agir de la projection de contours tridimensionnels sur un plan bidimensionnel: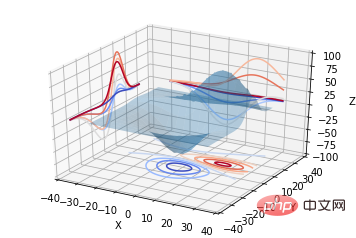

from mpl_toolkits.mplot3d import axes3d import matplotlib.pyplot as plt from matplotlib import cm fig = plt.figure() ax = fig.gca(projection='3d') X, Y, Z = axes3d.get_test_data(0.05) ax.plot_surface(X, Y, Z, rstride=8, cstride=8, alpha=0.3) cset = ax.contourf(X, Y, Z, zdir='z', offset=-100, cmap=cm.coolwarm) cset = ax.contourf(X, Y, Z, zdir='x', offset=-40, cmap=cm.coolwarm) cset = ax.contourf(X, Y, Z, zdir='y', offset=40, cmap=cm.coolwarm) ax.set_xlabel('X') ax.set_xlim(-40, 40) ax.set_ylabel('Y') ax.set_ylim(-40, 40) ax.set_zlabel('Z') ax.set_zlim(-100, 100) plt.show()

8. Diagrammes à barres (image)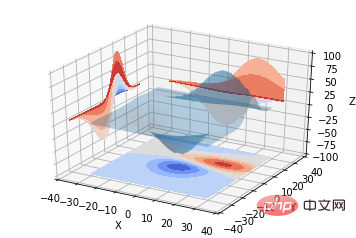

ax.bar(left, height, zs=0, zdir='z', *args, **kwargs

Copier après la connexion

x, y, zs = z, data

zdir : La direction de la planarisation du graphique à barres, qui peut être comprise en détail selon le code.
• code:

from mpl_toolkits.mplot3d import Axes3D import matplotlib.pyplot as plt import numpy as np fig = plt.figure() ax = fig.add_subplot(111, projection='3d') for c, z in zip(['r', 'g', 'b', 'y'], [30, 20, 10, 0]): xs = np.arange(20) ys = np.random.rand(20) # You can provide either a single color or an array. To demonstrate this, # the first bar of each set will be colored cyan. cs = [c] * len(xs) cs[0] = 'c' ax.bar(xs, ys, zs=z, zdir='y', color=cs, alpha=0.8) ax.set_xlabel('X') ax.set_ylabel('Y') ax.set_zlabel('Z') plt.show()

Copier après la connexion

9. Sous-intrigue (sous-intrigue)

from mpl_toolkits.mplot3d import Axes3D import numpy as np import matplotlib.pyplot as plt fig = plt.figure() ax = fig.gca(projection='3d') # Plot a sin curve using the x and y axes. x = np.linspace(0, 1, 100) y = np.sin(x * 2 * np.pi) / 2 + 0.5 ax.plot(x, y, zs=0, zdir='z', label='curve in (x,y)') # Plot scatterplot data (20 2D points per colour) on the x and z axes. colors = ('r', 'g', 'b', 'k') x = np.random.sample(20*len(colors)) y = np.random.sample(20*len(colors)) c_list = [] for c in colors: c_list.append([c]*20) # By using zdir='y', the y value of these points is fixed to the zs value 0 # and the (x,y) points are plotted on the x and z axes. ax.scatter(x, y, zs=0, zdir='y', c=c_list, label='points in (x,z)') # Make legend, set axes limits and labels ax.legend() ax.set_xlim(0, 1) ax.set_ylim(0, 1) ax.set_zlim(0, 1) ax.set_xlabel('X') ax.set_ylabel('Y') ax.set_zlabel('Z')

Copier après la connexion

Utilisation de la sous-intrigue B

MATLAB :

subplot(2,2,1) subplot(2,2,2) subplot(2,2,[3,4])

subplot(2,2,1) subplot(2,2,2) subplot(2,1,2)

import matplotlib.pyplot as plt from mpl_toolkits.mplot3d.axes3d import Axes3D, get_test_data from matplotlib import cm import numpy as np # set up a figure twice as wide as it is tall fig = plt.figure(figsize=plt.figaspect(0.5)) #=============== # First subplot #=============== # set up the axes for the first plot ax = fig.add_subplot(2, 2, 1, projection='3d') # plot a 3D surface like in the example mplot3d/surface3d_demo X = np.arange(-5, 5, 0.25) Y = np.arange(-5, 5, 0.25) X, Y = np.meshgrid(X, Y) R = np.sqrt(X**2 + Y**2) Z = np.sin(R) surf = ax.plot_surface(X, Y, Z, rstride=1, cstride=1, cmap=cm.coolwarm, linewidth=0, antialiased=False) ax.set_zlim(-1.01, 1.01) fig.colorbar(surf, shrink=0.5, aspect=10) #=============== # Second subplot #=============== # set up the axes for the second plot ax = fig.add_subplot(2,1,2, projection='3d') # plot a 3D wireframe like in the example mplot3d/wire3d_demo X, Y, Z = get_test_data(0.05) ax.plot_wireframe(X, Y, Z, rstride=10, cstride=10) plt.show()

Supplément :

from mpl_toolkits.mplot3d import Axes3D import matplotlib.pyplot as plt fig = plt.figure() ax = fig.gca(projection='3d') # Demo 1: zdir zdirs = (None, 'x', 'y', 'z', (1, 1, 0), (1, 1, 1)) xs = (1, 4, 4, 9, 4, 1) ys = (2, 5, 8, 10, 1, 2) zs = (10, 3, 8, 9, 1, 8) for zdir, x, y, z in zip(zdirs, xs, ys, zs): label = '(%d, %d, %d), dir=%s' % (x, y, z, zdir) ax.text(x, y, z, label, zdir) # Demo 2: color ax.text(9, 0, 0, "red", color='red') # Demo 3: text2D # Placement 0, 0 would be the bottom left, 1, 1 would be the top right. ax.text2D(0.05, 0.95, "2D Text", transform=ax.transAxes) # Tweaking display region and labels ax.set_xlim(0, 10) ax.set_ylim(0, 10) ax.set_zlim(0, 10) ax.set_xlabel('X axis') ax.set_ylabel('Y axis') ax.set_zlabel('Z axis') plt.show()

[Recommandations associées :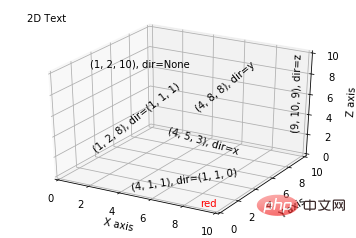Tutoriel vidéo Python3

Ce qui précède est le contenu détaillé de. pour plus d'informations, suivez d'autres articles connexes sur le site Web de PHP en chinois!