All Ancestors of a Node in a Directed Acyclic Graph

2192. All Ancestors of a Node in a Directed Acyclic Graph

Medium

You are given a positive integer n representing the number of nodes of a Directed Acyclic Graph (DAG). The nodes are numbered from 0 to n - 1 (inclusive).

You are also given a 2D integer array edges, where edges[i] = [from_i, to_i] denotes that there is a unidirectional edge from from_i to to_i in the graph.

Return a list answer, where answer[i] is the list of ancestors of the i^th node, sorted in ascending order.

A node u is an ancestor of another node v if u can reach v via a set of edges.

Example 1:

• Input: n = 8, edgeList = [[0,3],[0,4],[1,3],[2,4],[2,7],[3,5],[3,6],[3,7],[4,6]]
• Output: [[],[],[],[0,1],[0,2],[0,1,3],[0,1,2,3,4],[0,1,2,3]]
• Explanation: The above diagram represents the input graph.
  • Nodes 0, 1, and 2 do not have any ancestors.
  • Node 3 has two ancestors 0 and 1.
  • Node 4 has two ancestors 0 and 2.
  • Node 5 has three ancestors 0, 1, and 3.
  • Node 6 has five ancestors 0, 1, 2, 3, and 4.
  • Node 7 has four ancestors 0, 1, 2, and 3.

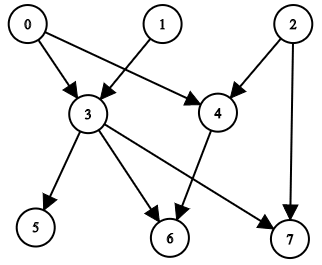
Example 2:

• Input: n = 5, edgeList = [[0,1],[0,2],[0,3],[0,4],[1,2],[1,3],[1,4],[2,3],[2,4],[3,4]]
• Output: [[],[0],[0,1],[0,1,2],[0,1,2,3]]
• Explanation: The above diagram represents the input graph.
  • Node 0 does not have any ancestor.
  • Node 1 has one ancestor 0.
  • Node 2 has two ancestors 0 and 1.
  • Node 3 has three ancestors 0, 1, and 2.
  • Node 4 has four ancestors 0, 1, 2, and 3.

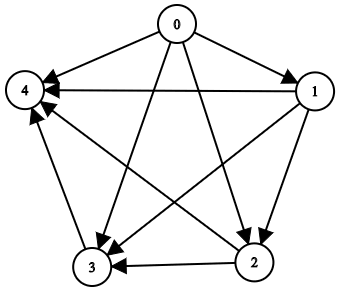
Constraints:

• 1 <= n <= 1000
• 0 <= edges.length <= min(2000, n * (n - 1) / 2)
• edges[i].length == 2
• 0 <= from_i, to_i <= n - 1
• from_i != to_i
• There are no duplicate edges.
• The graph is directed and acyclic.

Solution:

class Solution {

    /**
     * @param Integer $n
     * @param Integer[][] $edges
     * @return Integer[][]
     */
    function getAncestors($n, $edges) {
        $adjacencyList = array_fill(0, $n, []);
        foreach ($edges as $edge) {
            $from = $edge[0];
            $to = $edge[1];
            $adjacencyList[$to][] = $from;
        }

        $ancestorsList = [];

        for ($i = 0; $i < $n; $i++) {
            $ancestors = [];
            $visited = [];
            $this->findChildren($i, $adjacencyList, $visited);
            for ($node = 0; $node < $n; $node++) {
                if ($node == $i) continue;
                if (in_array($node, $visited))
                    $ancestors[] = $node;
            }
            $ancestorsList[] = $ancestors;
        }

        return $ancestorsList;
    }

    private function findChildren($currentNode, &$adjacencyList, &$visitedNodes) {
        $visitedNodes[] = $currentNode;
        foreach ($adjacencyList[$currentNode] as $neighbour) {
            if (!in_array($neighbour, $visitedNodes)) {
                $this->findChildren($neighbour, $adjacencyList, $visitedNodes);
            }
        }
    }
}




Contact Links


LinkedIn
GitHub



          

            
  

            
        
The above is the detailed content of All Ancestors of a Node in a Directed Acyclic Graph. For more information, please follow other related articles on the PHP Chinese website!